こんにちは。相城です。さて2020年度3月10日に行われた徳島県の高校入試の問題からです。それではどうぞ。
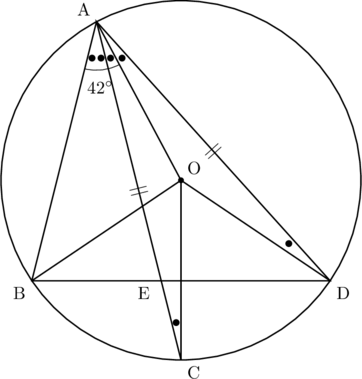
下の図のように、半径が15cmの円Oの周上に4点A、B、C、Dがあり、AC=ADである。また、弦ACは![]() の二等分線であり、弦ACと弦BDの交点をEとする。(1)~(3)に答えなさい。ただし、円周率は
の二等分線であり、弦ACと弦BDの交点をEとする。(1)~(3)に答えなさい。ただし、円周率は![]() とします。
とします。
(1) ![]() のとき、(a)、(b)に答えなさい。
のとき、(a)、(b)に答えなさい。
(a) ![]() の大きさを求めなさい。
の大きさを求めなさい。
(b) 点Aを含まないおうぎ形OBCの面積を求めなさい。
(2) △ABC![]() △AEDを証明しなさい。
△AEDを証明しなさい。
(3) 点Cを含まない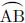 の長さが
の長さが![]() cmのとき、点Bを含まない弧ADの長さを求めなさい。
cmのとき、点Bを含まない弧ADの長さを求めなさい。

答え
(1)
(a)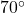
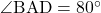 とする。CDを結ぶと△ACDが頂角
とする。CDを結ぶと△ACDが頂角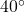 、底角
、底角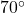 の二等辺三角形になり、
の二等辺三角形になり、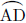 に対する円周角より、
に対する円周角より、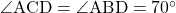
(b) cm
cm

よって、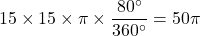
 cm
cm
(2)
△ABCと△AEDで、
仮定より
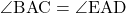 ・・・①
・・・①
AC AD・・・②
AD・・・②
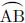 に対する円周角は等しいので、
に対する円周角は等しいので、
 ・・・③
・・・③
①、②、③より1組の辺とその両端の角がそれぞれ等しいので、
△ABC △AED
△AED
(3)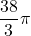 cm
cm
AとO、BとO、DとOを結ぶ。
中心角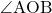 は
は
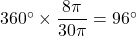
このとき、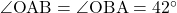
△OAC △OADとなり
△OADとなり とおくと、
とおくと、 より、
より、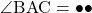 。このとき、
。このとき、 であるから、
であるから、 。よって、
。よって、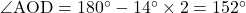
ゆえに、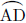
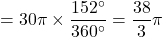
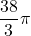 cm
cm
(a)
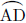 に対する円周角より、
に対する円周角より、(b)
よって、
(2)
△ABCと△AEDで、
仮定より
AC
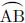 に対する円周角は等しいので、
に対する円周角は等しいので、①、②、③より1組の辺とその両端の角がそれぞれ等しいので、
△ABC
(3)
AとO、BとO、DとOを結ぶ。
中心角
このとき、
△OAC
ゆえに、
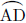
 数樂管理人
数樂管理人
この解き方以外でも解くことが可能です。以下のリンクからご覧ください。皆はどの解き方かな?
 TikZ:2020年度徳島県・こんなところに連立方程式の問題が
TikZ:2020年度徳島県・こんなところに連立方程式の問題が
 数樂管理人のブログ
数樂管理人のブログ 

