中学数学：円錐関連の公式の導出

2020年3月26日2022年5月29日

SHARE

こんにちは。相城です。円錐関連の公式の導出を書いておきます。単純に公式を暗記するのではなく, 覚えられる方は理屈も確認しておきましょう。

円錐の公式の導き方

円錐の展開図において, 側面のおうぎ形の半径(円錐の母線)を $R$ ,
側面のおうぎ形の弧の長さ(底面の円の周の長さ)を $\ell$ , おうぎ形の中心角を $a$ , 円錐の側面積(おうぎ形の面積)を $S$ , 底面の円の半径を $r$ とする。

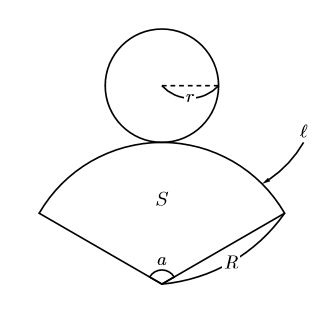
側面の扇形の弧の長さ $\ell$

$\ell=2\pi R\times\dfrac{a}{360}\cdots\textcircled{\scriptsize 1}$

側面の扇形の面積

$S=\pi R^2\times\dfrac{a}{360}\cdots\textcircled{\scriptsize 2}$

$\textcircled{\scriptsize 2}$ の式の両辺を2倍すると,

$2S=2\pi R^2\times\dfrac{a}{360}$

$R^2$ を $R\times R$ に分解して変形すると,

$2S=\underline{2\pi R\times\dfrac{a}{360}}\times R$

下線部は $\textcircled{\scriptsize 1}$ と同じ式であるから,

$2S=\ell\times R$

これより,

$S=\dfrac{1}{2}\ell R\cdots\textcircled{\scriptsize 3}$

また $\ell$ は円錐の底面の円周と等しいので,

$\ell =2\pi r\cdots\textcircled{\scriptsize 4}$

$\textcircled{\scriptsize 3}, \textcircled{\scriptsize 4}$ より,

$S=\dfrac{1}{2}R\times 2\pi r=\pi rR\cdots\textcircled{\scriptsize 5}$

$\textcircled{\scriptsize 1}$ を変形すると,

$\dfrac{a}{360}=\dfrac{\ell}{2\pi R}$

$\ell=2\pi r$ より,

$\dfrac{a}{360}=\dfrac{r}{R}$

よって,

$a=\dfrac{r}{R}\times360\cdots\textcircled{\scriptsize 6}$

まとめ

まとめ：円錐関連の公式

$R=$ 母線, $r=$ 底面の半径, $a=$ 側面の扇形の中心角として,

円錐の側面積 $S$ の公式
$S=\pi rR$
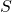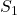円錐の表面積 $S_1$ の公式
(表面積) $=$ (側面積)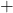 $+$ (底面積)
$S_1=S+\pi r^2=\pi rR+\pi r^2$
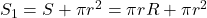
側面のおうぎ形の中心角 $a$
$a=\dfrac{r}{R}\times360^{\circ}$

$S$ に関しては $S=\dfrac12 \ell r$ という公式があるが, 登場は $S=\pi rR$ の方が使用頻度は高いと思われるので割愛している。では例題をやってみよう。

例題をやってみよう

下の図のような円すいの展開図がある。側面の展開図は, 半径が6cm, 中心角が
$a$ のおうぎ形で, 底面の円の半径は4cmである。このとき, (1), (2)の問いに答えなさい。ただし, 円周率は $\pi$ とする。

(1) 側面のおうぎ形の中心角 $a$ の大きさを求めなさい。
(2) 円すいの表面積を求めなさい。

(1)であるが,

$a=\dfrac{r}{R}\times360^{\circ}$

を用いて,

$a=\dfrac{4}{6}\times360^{\circ}=\dfrac23\times360^{\circ}=240^{\circ}$

(2)は, 側面積 $S$ を公式

$S=\pi rR$

を用いて,

$S=\pi\times4\times6=24\pi$

この $S$ に底面積 $\pi r^2=16\pi$ を加えて, 表面積 $S_1$ は,

$S_1=24\pi+16\pi=40\pi$

40 $\pi$ cm $^2$ となる。

プリントアウト用pdf

コメントを残すコメントをキャンセル

前の記事

中学数学：錐体は角錐の3分の1の体験(工作)

次の記事

中学数学：3等分線を考える