こんにちは。数IIIの極限のところで出てくる有名な公式です。これまた有名な証明方法で示していきましょう。最後にグラフの概形を載せています。
公式
![]()
以下の図形を用いて証明を行っていきます。それぞれの図形は, ![]() 頂角が
頂角が![]() , 2辺が1の二等辺三角形,
, 2辺が1の二等辺三角形,
扇形![]() 半径1,中心角
半径1,中心角![]() ,
, ![]() 直角を挟む2辺が,
直角を挟む2辺が, ![]() の直角三角形
の直角三角形
となっており, これらの大小関係を不等式で表して, 極限をとっていきます。

上の図において, 面積の関係から下の不等式が成立する。
ここで, それぞれ面積を求めると,
扇形
よって, 先の不等式にこれらを代入すると,
辺々を
辺々の逆数をとると,
ここで,
とすると,
また,
以上より,
が成り立つ。
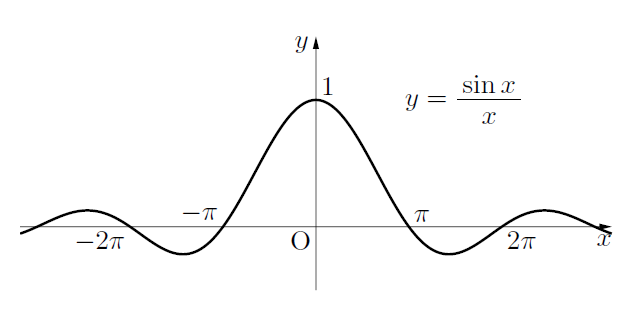
![]() のグラフの概形は以下のようになります。試験ではほとんど出てきませんので, 参考までに。大学で学ぶフーリエ変換とかのところでよく出てくるかもしれませんね?グラフはemathで描きました。縦軸と横軸のスケールは変更しています。
のグラフの概形は以下のようになります。試験ではほとんど出てきませんので, 参考までに。大学で学ぶフーリエ変換とかのところでよく出てくるかもしれませんね?グラフはemathで描きました。縦軸と横軸のスケールは変更しています。
 数樂管理人のブログ
数樂管理人のブログ