TikZ：高校数学：数III積分：斜回転体の体積について

こんにちは。これもよく登場する $xy$ 平面上の傾きのある直線を軸とする回転体の求め方の話です。先ずは例題を解きながら見ていきましょう。

例題

【例題】放物線 $y=x^2$ と直線 $y=x$ で囲まれた図形を, 直線 $y=x$ を軸として1回転させてできる立体の体積を求めよ。

図示すると以下のようになる。

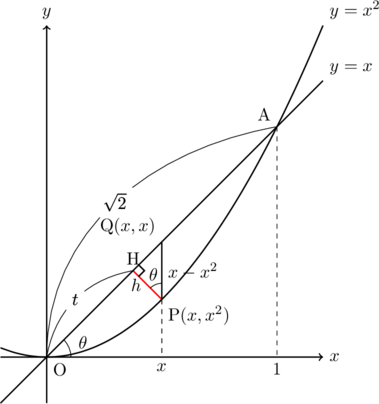
今回図中の $\theta$ (直線 $y=x$ と $x$ 軸とのなす角)は $45\Deg$ で, Pは放物線上の点, Qは $y=x$ 上の点で, $x$ 座標は等しい。 $\mathrm{P}( x, x^2)$ , $\mathrm{Q}( x, x )$ とする。このとき, 線分 $\mathrm{PH}$ ( $=h$ )を直線 $y=x$ を軸として1回転してできる円の面積を $y=x$ にそって線分 $\mathrm{OA}$ の区間で積分することを考える。 $\mathrm{OH}=t$ とすると, 求める体積 $V$ は,
$V=\pi\displaystyle\int_0^{\sqrt2}h^2\, dt\cdots\maru1$
と表せる。
ここで, $h,\ t$ を求めると,
$h=\mathrm{PQ}\cos 45\Deg$ , $\mathrm{PQ}=x-x^2$ なので,
$h=\dfrac{x-x^2}{\sqrt2}\cdots\maru2$
$t=\mathrm{OQ}-\mathrm{QH}$ であり, $\mathrm{OQ}=\sqrt2 x$ , $\mathrm{QH}=\mathrm{PH}(h)=\dfrac{x-x^2}{\sqrt2}$ であるから,
$t=\sqrt2 x-\dfrac{x-x^2}{\sqrt2}=\dfrac{x+x^2}{\sqrt2}$
この $t$ を $x$ で微分すると,
$dt=\dfrac{1+2x}{\sqrt2}\ dx\cdots\maru3$
となる。 $\maru2, \maru3$ より, $\maru1$ を計算すると,
$\begin{array}{lll}V&=&\pi\displaystyle\int_0^{\sqrt2}h^2\ dt\\&=&\pi\displaystyle\int_0^1\left(\dfrac{x-x^2}{\sqrt2}\right)^2\dfrac{1+2x}{\sqrt2}\ dx\\&=&\dfrac{\pi}{2\sqrt2}\displaystyle\int_0^1(x^2-3x^4+2x^5)\ dx\\&=&\dfrac{\pi}{2\sqrt2}\left[\dfrac13x^3-\dfrac35x^5+\dfrac13x^6\right]_0^1\\&=&\dfrac{\pi}{30\sqrt2}\end{array}$
となる。

一般化すると

関数 $y=f(x)$ と直線 $y=g(x)$ によって囲まれる面積を, 直線 $y=g(x)$ について1回転させてできる立体の体積を求めてみる。2つのグラフの交点の $x$ 座標は $x_1, x_2\ (x_1<x_2)$ とする。

直線 $y=g(x)$ と $x$ 軸のなす角を $\theta$ とする。Pは関数 $y=f(x)$ 上の点, Qは $y=g(x)$ 上の点で, $x$ 座標は等しい。 $\mathrm{P}( x, f(x) )$ , $\mathrm{Q}( x, g(x) )$ とする。このとき, 線分 $\mathrm{PH}$ ( $=h$ )を直線 $y=g(x)$ を軸として1回転してできる円の面積を $y=g(x)$ にそって線分 $\mathrm{A'A}$ の区間で積分することを考える。 $\mathrm{OH}=t$ とすると, 求める体積 $V$ は,
$V=\pi\displaystyle\int_{x_1}^{x_1+T}h^2\, dt\cdots\maru1$
と表せる。
ここで, $h,\ t$ を求めると,
$h=\mathrm{PQ}\cos\theta$ , $\mathrm{PQ}=g(x)-f(x)=h(x)$ とおくと,
$h=h(x)\cos\theta\cdots\maru2$
$t=\mathrm{OQ}-\mathrm{QH}$ であり, $\mathrm{OQ}=\dfrac{x}{\cos\theta}$ , $\mathrm{QH}=h(x)\sin\theta$ であるから,
$t=\dfrac{x}{\cos\theta}-h(x)\sin\theta$
この $t$ を $x$ で微分すると,
$dt=\dfrac{1}{\cos\theta}-h'(x)\sin\theta\ dx\cdots\maru3$
となる。 $\maru2, \maru3$ より, $\maru1$ を計算すると,
$\begin{array}{lll}V&=&\pi\displaystyle\int_{x_1}^{x_1+T}h^2\ dt\\&=&\pi\displaystyle\int_{x_1}^{x_2}\left\{h(x)\cos\theta\right\}^2\left\{\dfrac{1}{\cos\theta}-h'(x)\sin\theta\right\}\ dx\\&=&\pi\displaystyle\int_{x_1}^{x_2}\left\{(h(x))^2\cos\theta-(h(x))^2 h'(x)\sin\theta\cos^2\theta\right\}\ dx\\&=&\pi\cos\theta\displaystyle\int_{x_1}^{x_2}\left\{h(x)\right\}^2\ dx-\pi\sin\theta\cos^2\theta\displaystyle\int_{x_1}^{x_2}\left\{h(x)\right\}^2h'(x)\ dx\\&=&\pi\cos\theta\displaystyle\int_{x_1}^{x_2}\left\{h(x)\right\}^2\ dx-\pi\sin\theta\cos^2\theta\left[\dfrac{\left\{h(x)\right\}^3}{3}\right]_{x_1}^{x_2}\cdots\textcircled{\scriptsize 4}\\&=&\pi\cos\theta\displaystyle\int_{x_1}^{x_2}\left\{h(x)\right\}^2\ dx\end{array}$
となる。
よって, 求める体積は
$V=\pi\cos\theta\displaystyle\int_{x_1}^{x_2}\left\{h(x)\right\}^2\ dx$
で与えられる。
ちなみに $\textcircled{\scriptsize 4}$ の $\left[\dfrac{\left\{h(x)\right\}^3}{3}\right]_{x_1}^{x_2}$ の計算であるが,
$h(x)$ はもともと $g(x)-f(x)$ であり, $x_1, x_2$ は $f(x), g(x)$ の交点であるため, $f(x_1)=g(x_1)$ , $f(x_2)=g(x_2)$ となるので, 計算結果は0になる。
また, 関数 $y=f(x)$ と $y=g(x)$ の上下が逆転するときも, 同様にして求められる。具体的に書くと,
$t=\dfrac{x}{\cos\theta}+h(x)\sin\theta$
とすることで, 同じ結果が得られる。

例題

一般化すると

コメントを残す コメントをキャンセル

コメントを残すコメントをキャンセル